
Kansas Geological Survey, Bulletin 134, Part 6, originally published in 1959

Originally published in 1959 as Kansas Geological Survey Bulletin 134, Part 6. This is, in general, the original text as published. The information has not been updated.
Dip bearings of water-deposited cross-strata in sandstone of the Dakota Formation (Cretaceous) of central Kansas were measured at 79 localities in Ottawa County. Vector-resultant (average) dip bearings lie in nearly all compass directions, but average S 57° W, showing that transportation of Dakota sediments in Ottawa County was mainly from northeast to southwest and that the source, therefore, seemingly lay to the northeast. Computation of a regression line of standard deviation on consistency ratio indicates that, in Dakota sandstone, consistency ratio is a good predictor of standard deviation for ratio values between 0.65 and 0.95.
The Dakota Formation (Cretaceous) of Kansas is a source of clay for a large and growing ceramic industry, of water for farms, municipalities, and industry, and of construction materials. Subsurface equivalents of the Dakota Formation seemingly have potentiality for oil production (Merriam, 1958). As part of a program to supplement stratigraphic information on the economically important Dakota Formation, studies of cross-stratification have been started in Ottawa, Ellsworth, and other counties.
This report presents results of studies of cross-stratification in sandstone of the Dakota Formation in Ottawa County, which is in the approximate middle of the belt of Dakota outcrops in central Kansas (Fig. 1). Cross-stratification dip bearings (directions of dip of cross-strata) show direction of transport of sediments or direction of flow of currents that deposited them. In conjunction with other geologic data, cross-stratification dip bearings may be useful in determination of the source of Dakota sediments, the extent of Dakota sedimentation, and the environment in which the Dakota rocks were deposited, and thereby may be useful in delimiting those areas of Dakota rocks or their subsurface equivalents that have value as a source of clay, ground-water, and petroleum and natural gas. It is hoped that the information in this report will supplement that being gained by a geologic mapping and ground-water study of Ottawa County being made by L.E. Mack.
Figure 1--Index map showing location of Ottawa County and outcrop areas of Dakota Formation, Kansas.

Many readers may not be interested in the statistical methods used in this study of cross-stratification, but statements of the methods employed and the mathematical relations used in computation of results are included, not only for completeness, but also for those readers interested in statistical treatment of geologic data. Detailed descriptions and discussions applicable to statistical analysis of cross-strata and other geologic data are found in Reiche (1938), Curray (1956), and Pincus (1956).
The general stratigraphy of the Dakota Formation has been described by Plummer and Romary (1942), and various special features have been studied by several workers: cementation of some Dakota sandstone (Swineford, 1947); clay in the Dakota Formation (Plummer and Romary, 1947; Plummer and others, 1954); coal resources of the Dakota Formation (Schoewe, 1952); and subsurface extensions of the Dakota Formation (Merriam, 1957; Merriam and others, 1959). Rubey and Bass (1925, p. 59) studied cross-stratification in conjunction with their work on the Rocktown channel sandstone in Russell County. Tester (1931, p. 262, 267, and 270) mentioned quadrants in which cross-strata dip at localities in Washington, Rice, and Ellsworth Counties, Kansas. Twenhofel (1924, p. 39) stated that cross-strata dip in a southerly direction but did not specify whether he referred to the Dakota Formation alone or to all sandstone in the pre-Graneros Cretaceous of Kansas.
The writers thank Floyd W. Preston of the State Geological Survey and the Department of Petroleum Engineering of the University of Kansas who programmed the cross-stratification dip bearings for computation of standard deviation on the University's I.B.M. 650 computer. Leslie E. Mack kindly provided information on the Dakota Formation from a study, in progress, of the geology and ground-water resources of Ottawa County.
In Ottawa County, the Dakota Formation (Gulfian) is overlain conformably by the Graneros Shale, rests with gently undulating disconformity on the Kiowa Shale (Comanchean), and overlaps redbeds of the Wellington Formation (Permian). Relief on the Kiowa-Dakota contact locally measures as much as 50 feet; relief on the Permian-Cretaceous boundary measures as much as 75 feet (Mack, L.E., 1959, oral communication). Stratigraphic thickness of the Dakota Formation approximates 300 feet (Mack, L.E., 1959, oral communication). Cretaceous rocks younger than the Dakota Formation are restricted to the northwest part of Ottawa County, whereas Kiowa and Wellington beds are exposed in the southeast part of the county (Plate 1).
The Dakota Formation is divided into the Janssen Clay member and the underlying Terra Cotta Clay member (Plummer and Romary, 1942). The Terra Cotta Clay includes "massive clay, silt, and sandstone comprising approximately the lower two-thirds of the Dakota formation. The most conspicuous lithological feature of the Terra Cotta member is the wide-spread distribution of gray-and-red mottled massive clay. The Janssen member includes beds of lignite, gray to dark-gray massive clay, silt, and some shale . . ." (Plummer and Romary, 1942, p. 319). Although both members are present in Ottawa County, mapping of their contact is virtually impossible, owing to the gradational nature of the contact, to incorporation of dark claystone in parts of the Terra Cotta, and to incorporation of light-colored mottled claystone in the Janssen.
The Dakota Formation of Ottawa County is composed of 60 to 70 percent claystone, mudstone, and siltstone, and 30 to 40 percent sandstone. The argillaceous rocks range from very light gray to yellowish and reddish gray, reddish purple, and dark gray. Dark gray dominates in the upper one-third of the formation but also is common in the basal part of the formation, within 30 feet of the Kiowa-Dakota contact. For the most part, the claystone and mudstone are massive, but within the Janssen member and along the Kiowa-Dakota contact they locally are laminated to thin laminated (McKee and Weir, 1953, p. 383). Although the argillaceous rocks contain various amounts of iron oxides and sparse montmorillonite, they are composed mainly of kaolinite (Plummer and Romary, 1947, p. 38) and a lesser amount of illite. X-ray diffraction studies of clay samples taken near the Kiowa-Dakota contact indicate that claystone containing abundant illite is intercalated in the lower parts of the Dakota Formation as sparse medium- to dark-gray locally fissile beds that bear superficial resemblance to Kiowa shales.
Sandstone in the Dakota Formation of Ottawa County is light gray, very pale and dark yellowish orange, and reddish brown. It occurs as beds less than 1 foot thick and as massive lenses as much as 60 feet thick and extending as far as 6 miles laterally. Sandstone lenses and layers locally occupy scour channels in underlying claystone and mudstone. Elsewhere, sandstone bodies interfinger laterally with the surrounding argillaceous rocks. Bedding is thin to thick (McKee and Weir, 1953, p. 383), and cross-stratification is abundant.
Most Dakota sandstone is medium fine grained, moderately well rounded, and well sorted. Rounding, however, commonly is masked by etching of the grains and by quartz overgrowths. Sieve analyses of 13 grab samples of sandstone from Ottawa County indicate that median grain diameters range from 0.93Ø (0.54 mm) to 3.06Ø (0.12 mm) and have a mean at 2.27Ø (0.21 mm). The mean quartile deviation for the 13 samples averages 0.25Ø. Quartz is the major mineral constituent; minor amounts (less than 5 percent) of mica and feldspar (both sodic and potassic) and traces of various heavv minerals (tourmaline, zircon, leucoxene, etc.) also are present. Locally the sandstone contains grains of "limonite" that measures as much as 1 mm in diameter and probably are altered from secondary siderite. Clay and "limonite" are the major constituents of the matrix, which commonly is not as much as 5 percent of the rock.
Most of the sandstone is friable and lightly stained by iron oxide. Commonly, however, abundant iron oxide cement is present, almost to the exclusion of quartz grains, where sandstone beds cap hills and benches. Where abundant iron oxide has accumulated in sandstone, bedding is masked by Liesegang rings, pipe-like structures (Pl. 2A), and cellular pitting (Pl. 2B). Thorp and Reed (1949, p. 69) examined cellular iron oxide accumulations in the Dakota Group of Nebraska and concluded that they represent a Cretaceous lateritic subsoil. Subsurface studies by Mack (1959, oral communication) and surface studies by the authors indicate that concentration of iron oxide in Dakota sandstone in Ottawa County is a feature of present topography that seemingly relates to length of exposure to weathering. Earlier work by Swineford (1947, p. 71), Plummer and Romary (1942, p. 327), and Rubey and Bass (1925, p. 57) also indicates that much of the iron cementation in Dakota sandstone is case-hardening.
Plate 2--Photographs of Dakota sandstone, Ottawa County, Kansas. A., iron oxide enriched sandstone showing pipe-like structures, sec. 12, T. 11 S., R. 3 W.; scale is 6.5 inches long. B., iron oxide enriched sandstone showing cellular structure, sec. 17, T. 10 S., R. 1 W. C., tabular cross-stratified sandstone, sec. 34, T. 9 S., R. 2 W. D., same as C: scale is 6.5 inches long. E., lenticular cross-stratified sandstone, sec. 32, T. 11 S., R. 3 W. F., cross-stratified calcite-cemented sandstone concretions showing both tabular and wedge-shaped planar sets, "Rock City", sec. 14, T. 11 S., R. 4 W.
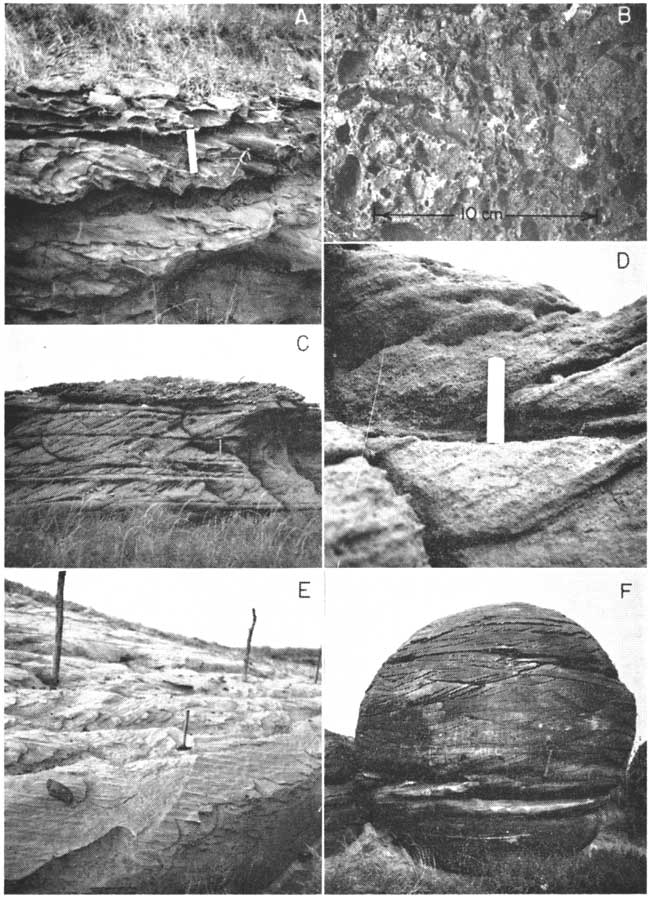
Calcite also is a common cement in Dakota sandstone in Ottawa County. At "Rock City" (S2 sec. 14, T. 11 S., R. 4 W.) and in the SE sec. 20, T. 9 S., R. 2 W., large concretionary masses of calcite-cemented sandstone are found. Similarly cemented sandstone occurs locally elsewhere in Ottawa County. Schoewe and others (1937) described the distribution of the concretions at "Rock City", and Swineford (1947, p. 76, 82) described the cement and its probable origin.
Dakota sediments are thought to be nonmarine and to have been deposited "near sea-level" over a wide area (Plummer and Romary, 1942, p. 342, 344). According to Tester (1931, p. 280), the sediments of the Dakota were ". . . laid down in an environment at and near the shore line of a broad, relatively shallow ocean. The material was derived from the north and northeast and was carried southward by streams of small competency which may have had wide flood plains and which when reaching the coastline built wide and ramifying deltas. At times these deltas extended themselves seaward with a development of bar-like fingers of relatively coarse material, with the minor distributaries of the streams throwing off the finer silt and clays which were deposited on the flank of the principal distributary." The near-sealevel environment of Dakota sedimentation is thought to have persisted throughout Dakota time (Plummer and Romary, 1942, p. 344). The abundance of lignite and black lignitic clay near the top of the Dakota Formation is interpreted as indicating swampy conditions that heralded deposition of the overlying marine Graneros Shale, which rests conformably on the Dakota (Plummer and Romary, 1942, p. 344.)
Description
The Dakota sandstone of Ottawa County shows each of the major classes of cross-stratification defined by McKee and Weir (1953). Planar and lenticular sets, however, are much more abundant than simple cross-stratification. Planar cross-stratification, which is most common, includes abundant tabular sets (Pl. 2C, D) and sparse wedge-shaped sets. Within the sets, the cross-strata are medium and small scale, thin laminated to thin bedded, high angle, most commonly straight in longitudinal section, and mainly concave in plan. Lenticular cross-stratification is virtually all of the medium-scale trough type (Pl. 2E) in which individual cross-strata are thin laminated to thin bedded, both high and low angle, and concave in both section and plan. At most exposures, only one type of cross-stratification can be seen; at others, however, planar cross-stratification may overlie trough, or wedge-shaped sets may be intercalated with tabular sets (Pl. 2F).
Field Methods
Field work was done during the summer of 1958. An attempt was made to measure cross-strata dip bearings at sandstone exposures approximately 2 miles apart in order to have uniform areal sampling, but distances between localities studied range from 1 to 5 miles, depending on the abundance of cross-stratified sandstone outcrops. In total, cross-strata dip bearings were measured at 79 localities in an area of approximately 660 square miles underlain by the Dakota Formation. In much of the area, however, rocks of the Dakota Formation are concealed by undifferentiated Pleistocene and Recent deposits.
Cross-strata dip bearings were measured with a Brunton pocket transit and grouped in 5-degree class intervals. In general, an attempt was made to take one observation per set of cross-strata exposed at each locality. The number of measurements recorded at each locality ranged from 5 to 80, depending upon the size and abundance of outcrops and the degree to which they showed cross-stratification. Actual measurement of dip bearings was done by Franks and Coleman, who worked together. Accordingly, operator error should be minimal.
The maximum number of observations made at exposures showing abundant sets of cross-strata was determined by adaptation of a method outlined by Raup and Miesch (1957), who used a graphical estimate of 2 standard deviations based on 50 observations to approximate a flatness point (Reiche, 1938). Instead of a base of 50 measurements, a base of 25 was used because of the comparative paucity of cross-stratified sets.
Statistical Treatment of Data
Cross-strata dip bearings, which had been grouped in the field to 5-degree class intervals, were grouped further to 10-degree class intervals, and vector-resultant dip bearings were computed as average dip bearings for each locality by methods similar to those outlined by Pincus (1956, p. 544) and Curray (1956, p. 118). The direction of the vector resultant is given by:
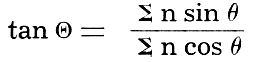
where Θ is the vector-resultant dip bearing in degrees, θ the midpoint of each class interval, and n the class frequency (Curray, 1956, p. 119). For purposes of calculation, we assigned each cross-strata dip bearing a vector length of unity rather than prorating the vector lengths by the amount of dip of each cross-stratum.
As a measure of dispersion of cross-strata dip bearings, Reiche (1938, p. 913) defined the "consistency ratio," which is the length of the vector resultant (Θ) divided by the sum of the vector lengths of each cross-stratum dip bearing. The consistency ratio can be computed by:
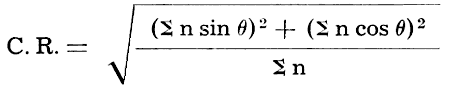
(Curray, 1956, p. 119), and it ranges from 0 to 1 (0 to 100 percent). "A random distribution of orientations will give a magnitude of 0 percent . . ." and perfect orientation gives a magnitude of 100 percent (Curray, 1956, p. 120). Further, according to Curray (1956, p. 120), the consistency ratio is a sensitive measure of dispersion.
Standard deviation of cross-strata dip bearings can be calculated by use of the vector-resultant dip bearing as a mean (Curray, 1956, p. 122, 124; Pincus, 1956, p. 554). The standard deviation of data recorded at each locality was computed according to the equation:
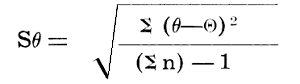
where Sθ is the standard deviation, (θ - Θ) is the difference between each cell midpoint in degrees and the bearing of the vector resultant in degrees, and Σn is the sum of the class frequencies or the total number of measurements made at a locality.
The number of observations, the vector-resultant dip bearing, consistency ratio, and one standard deviation are given in Table 1 for each of the 79 localities where cross-strata dip bearings were measured. Figure 2 is a scatter diagram on which the standard deviation at each locality is plotted against the consistency ratio. The least-squares regression line and standard error lines also are plotted. The equation of the calculated regression line is:
y = 136.77 - 122.60x
where y is one standard deviation in degrees and x is the consistency ratio. One standard error is 5.3° and the correlation coefficient is 0.93. Seemingly, for large consistency ratios (0.65 to 0.95), the regression line can be used as a good predictor of standard deviation, although the consistency ratio and standard deviation do not have a straight-line relationship (Curray, 1956, p. 122; Pincus, 1956).
Figure 2--Scatter diagram of standard deviation on consistency ratio for each of 79 localities in Ottawa County where cross-strata dip bearings were measured. The regression line of standard deviation on consistency ratio is bounded by lines showing one standard error.
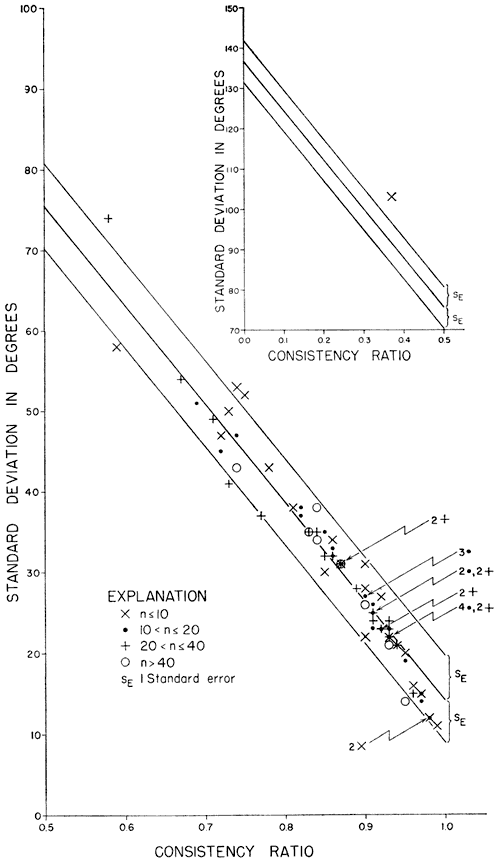
Table 1--Vector-resultant (average) dip bearings of cross-stratification in Dakota sandstone, Ottawa County, Kansas.
| Quadrant | Number of measurements |
Bearing of vector resultant |
Consistency ratio |
Standard deviation |
Outcrop location (section, township, range) |
|---|---|---|---|---|---|
| Northeast: | 7 | N 82° E | 0.37 | 103° | 11-10-5 |
| 12 | N 54° E | 0.86 | 32° | 33-10-1 | |
| 17 | N 53° E | 0.82 | 38° | 19-10-1 | |
| 20 | N 54° E | 0.92 | 23° | 13-11-2 | |
| 30 | N 70° E | 0.91 | 25° | 23-11-3 | |
| Southeast: | 6 | S 85° E | 0.95 | 20° | 12-10-2 |
| 6 | S 38° E | 0.90 | 31° | 28-12-5 | |
| 7 | S 67° E | 0.98 | 12° | 27-11-2 | |
| 7 | S 6° E | 0.90 | 28° | 23-12-3 | |
| 10 | S 88° E | 0.59 | 58° | 32-10-1 | |
| 11 | S 11° E | 0.90 | 27° | 35-11-5 | |
| 11 | S 5° E | 0.91 | 23° | 13-12-3 | |
| 14 | S 16° E | 0.69 | 51° | 2-11-1 | |
| 15 | S 63° E | 0.98 | 12° | 28-12-2 | |
| 15 | S 47° E | 0.93 | 22° | 5-11-2 | |
| 20 | S 49° E | 0.85 | 35° | 19-12-2 | |
| 20 | S 43° E | 0.72 | 45° | 29-10-2 | |
| 21 | S 13° E | 0.87 | 31° | 25-12-3 | |
| 25 | S 44° E | 0.89 | 28° | 8-10-4 | |
| 25 | S 31° E | 0.71 | 49° | 36-9-5 | |
| 25 | S 5° E | 0.85 | 32° | 8-12-3 | |
| 32 | S 6° E | 0.91 | 25° | 1-9-1 | |
| 40 | S 59° E | 0.92 | 23° | 17-11-2 | |
| Southwest: | 6 | S 53° W | 0.98 | 12° | 12-11-3 |
| 6 | S 56° W | 0.86 | 34° | 12-12-4 | |
| 7 | S 36° W | 0.99 | 11° | 10-11-2 | |
| 8 | S 53° W | 0.74 | 53° | 4-11-5 | |
| 9 | S 42° W | 0.93 | 22° | 20-10-4 | |
| 10 | S 32° W | 0.72 | 47° | 31-11-2 | |
| 10 | S 39° W | 0.87 | 31° | 34-9-5 | |
| 13 | S 22° W | 0.86 | 33° | 18-11-4 | |
| 14 | S 85° W | 0.82 | 37° | 36-10-2 | |
| 15 | S 23° W | 0.91 | 26° | 8-12-5 | |
| 16 | S 24° W | 0.93 | 23° | 13-10-5 | |
| 18 | S 19° W | 0.91 | 25° | 8-9-1 | |
| 18 | -W | 0.90 | 27° | 2-9-2 | |
| 19 | S 26° W | 0.93 | 22° | 3-9-1 | |
| 20 | S 41° W | 0.97 | 15° | 28-9-1 | |
| 20 | S 50° W | 0.93 | 22° | 11-9-1 | |
| 23 | S 59° W | 0.67 | 54° | 11-10-1 | |
| 25 | S 51° W | 0.83 | 35° | 19-12-5 | |
| 25 | S 56° W | 0.87 | 31° | 8-11-4 | |
| 26 | S 88° W | 0.91 | 24° | 34-11-4 | |
| 30 | S 6° W | 0.77 | 37° | 9-10-2 | |
| 30 | S 12° W | 0.58 | 74'° | 30-11-3 | |
| 30 | S 25° W | 0.93 | 22° | 15-9-1 | |
| 32 | S 55° W | 0.93 | 22° | 20-9-2 | |
| 32 | S 80° W | 0.93 | 24° | 16-10-2 | |
| 35 | S 19° W | 0.96 | 15° | 34-9-2 | |
| 40 | S 72° W | 0.86 | 32° | 21-11-4 | |
| 42 | S 35° W | 0.93 | 21° | 18-10-5 | |
| 50 | S 87° W | 0.84 | 34° | 15-9-2 | |
| 61 | S 30° W | 0.90 | 26° | 32-9-4 | |
| Northwest: | 5 | N 70° W | 0.92 | 27° | 19-12-4 |
| 5 | N 66° W | 0.85 | 35° | 2-11-2 | |
| 6 | N 63° W | 0.73 | 50° | 7-9-3 | |
| 7 | N 76° W | 0.78 | 43° | 19-10-4 | |
| 8 | N 45° W | 0.75 | 52° | 31-12-3 | |
| 9 | N 86° W | 0.96 | 16° | 34-12-3 | |
| 10 | N 87° W | 0.97 | 15° | 36-9-2 | |
| 10 | N 86° W | 0.90 | 27° | 1-12-5 | |
| 10 | N 80° W | 0.94 | 21° | 35-10-3 | |
| 10 | N 69° W | 0.81 | 38° | 4-11-3 | |
| 11 | N 34° W | 0.91 | 25° | 7-10-2 | |
| 12 | N 20° W | 0.97 | 14° | 25-11-4 | |
| 17 | N 54° W | 0.90 | 27° | 23-11-5 | |
| 18 | +W | 0.74 | 47° | 31-9-5 | |
| 19 | N 85° W | 0.95 | 19, | 25-9-4 | |
| 20 | N 70° W | 0.93 | 22° | 17-11-5 | |
| 22 | N 55° W | 0.93 | 23° | 9-10-3 | |
| 25 | N 40° W | 0.94 | 21° | 27-12-4 | |
| 25 | N 35° W | 0.78 | 41° | 31-9-2 | |
| 30 | N 62° W | 0.92 | 23° | 10-11-5 | |
| 33 | N 39° W | 0.84 | 35° | 2-10-3 | |
| 50 | N 78° W | 0.87 | 31° | 1-11-4 | |
| 50 | N 55° W | 0.95 | 19, | 31-10-5 | |
| 75 | N 78° W | 0.84 | 38° | 14-11-4 | |
| 80 | N 81° W | 0.83 | 35° | 32-11-3 | |
| 80 | N 44° W | 0.74 | 43° | 26-9-5 |
Direction of Transportation of Dakota Sandstone
The dip bearing of cross-strata is interpreted to be the direction of flow of currents that deposited the strata, and therefore the direction of transport of the sediments. The localities at which cross-strata dip bearings were measured are shown on Plate 1, as are the average directions of transportation (vector-resultant dip bearings), the number of individual dip bearings taken, and the standard deviation of the dip bearings.
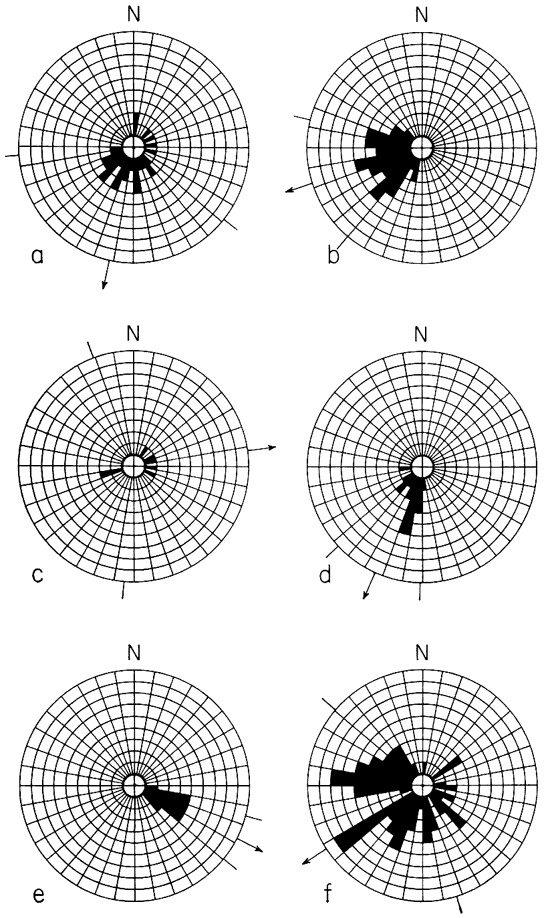
The differences in consistency ratio and standard deviation of cross-strata dip bearings indicate diversity in degree of preferred orientation from locality to locality. Large standard deviations and small consistency ratios in general indicate more random distribution, but standard deviation and consistency ratio locally are misleading. Comparison of the circular histograms of Figure 3, in which individual cross-strata dip bearings from five localities are plotted, shows that the data in Figure 3a have great dispersion, large standard deviation, and small consistency ratio. The data plotted in Figure 3b have moderately consistent preferred orientation, small standard deviation, and large consistency ratio. In contrast, the data shown in Figure 3c have moderate preferred orientation but large standard deviation and small consistency ratio, owing to the antipodal position of the cross-strata dip bearings. Figure 3d shows a distinctly skewed distribution of the dip bearings but has small standard deviation and large consistency ratio. Figure 3e has almost uniform preferred orientation and correspondingly very small standard deviation and a consistency ratio of almost unity.
Figure 3--a through e, circular histograms of cross-strata dip bearings showing differences in dispersion of data and standard deviation. Each block represents one measurement; arrow is vector resultant; arc between lines is one standard deviation plotted on each side of the vector resultant. a, sec. 30, T. 11 S., R. 3 W.; standard deviation, 74°; consistency ratio, 0.58. b, sec. 21, T. 10 S., R. 5 W.: standard deviation, 32°; consistency ratio, 0.86. c, sec. 11, T. 10 S., R. 5 W.; standard deviation 103°; consistency ratio, 0.37. d, sec. 13, T. 10 S., R. 5 W.; standard deviation, 23°; consistency ratio, 0.93. e, sec. 28, T. 12 S., R. 2 W.; standard deviation 12°; consistency ratio, 0.98. f, circular histogram of vector-resultant dip bearings for all localities in Ottawa County; each block is one vector resultant; arrow is average vector resultant; arc between lines, one standard deviation (74°) plotted each side of average vector resultant; consistency ratio, 0.43.
The map indicates considerable variation of average current direction in some areas (particularly in T. 10 and 11 S., R. 2 W., and in T. 10 S., R. 5 W.). Elsewhere on the map, as in T. 9 S., R. 1 W., the vector resultants show preferred orientation and similarity in standard deviation as well. Field studies show that many of the vector resultants that have about the same bearing are calculated from cross-strata dip bearings measured in different areal parts of the same sandstone body. Further, where the vector resultants have more diverse orientation, they were calculated mainly from cross-strata dip bearings in different sandstone bodies. In general, areas showing greatest diversity of vector-resultant dip bearings have greatest topographic relief.
Figure 3f is a circular histogram in which each of the 79 vector-resultant dip bearings is plotted. The distribution is bimodal, but the paucity of individual vector resultants between S 60° W and S 80° W may be in part the result of grouping at three different levels: first, grouping dip bearings in the field; second, grouping dip bearings for computation of individual vector resultants; and finally, grouping vector resultants to 10-degree class intervals for plotting of the diagram. The bimodal distribution also may reflect meandering or braiding of streams that deposited most Dakota sandstone. For the most part, directions of current flow in a braided or meandering stream will be at an angle to the over-all direction of flow, and only locally are current directions and the over-all trend likely to be coincident. The average direction of transport in Ottawa County computed by the equation
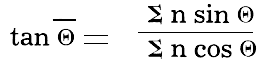
is S 57° W; the consistency ratio is 0.43; standard deviation is 74°.
Cross-stratification in Dakota sandstone of Ottawa County includes small- and medium-scale planar and lenticular sets. Planar is most common, and much of it is of high-angle tabular form in which strata dip more than 20°. Vector-resultant dip bearings lie in nearly all compass directions but average S 57° W. It is inferred that the currents that transported the Dakota sediments traveled mainly from northeast to southwest, and therefore that the source of the Dakota sediments in Ottawa County lay to the northeast. The diversity of vector-resultant dip bearings indicates considerable local variation in direction of transport.
The local variations in vector-resultant dip bearings lend support to the concept of Dakota sedimentation presented by Plummer and Romary (1942) and Tester (1931), who visualized deposition of Dakota sediments in a deltaic-coastal plain environment at the margins of a shallow sea. The vector-resultant dip bearings probably are no more diversely orientated than would be expected of braided and meandering streams (Hamblin, 1958, p. 52).
Computation of a least-squares regression line and standard error lines of standard deviation of cross-strata dip bearings on their consistency ratio shows that for ratios between 0.65 and 0.95 the consistency ratio is a good predictor of standard deviation. The correlation coefficient is 0.93.
Curray, J.R. (1956) Analysis of two-dimensional orientation data: Jour. Geol., v. 64, p. 117-131.
Hamblin, W.K. (1958) Cambrian sandstones of northern Michigan: Michigan Geol. Survey, pub. 51, p. 1-149.
McKee, E.D., and Weir, G.W. (1953) Terminology for stratification and cross-stratification in sedimentary rocks: Geol. Soc. America, Bull., v. 64, p. 381-390.
Merriam, D.F. (1957) Subsurface correlation and stratigraphic relation of rocks of Mesozoic age in Kansas: Kansas Geol. Survey, Oil and Gas Invest. 14, p. 1-25.
Merriam, D.F. (1958) Cretaceous possibilities good for northwest Kansas: Oil and Gas Jour., v. 56, no. 13, p. 138-139, 142.
Merriam, D.F., and others (1959) Description of a Dakota (Cretaceous) core from Cheyenne County, Kansas: Kansas Geol. Survey, Bull. 134, pt. 1, p. 1-104. [available online]
Pincus, H.J. (1956) Some vector and arithmetic operations on two-dimensional variates, with application to geologic data: Jour. Geol., v. 64, p. 533-537.
Plummer, Norman, and Romary, J.F. (1942) Stratigraphy of the pre-Greenhorn Cretaceous beds of Kansas: Kansas Geol. Survey, Bull. 41, pt. 9, p. 313-348. [available online]
Plummer, Norman, and Romary, J.F. (1947) Kansas clay, Dakota Formation: Kansas Geol. Survey, Bull. 67, p. 1-241.
Plummer, Norman, and others (1954) Chemical, petrographic, and ceramic properties of four clays from the Dakota Formation in Kansas: Kansas Geol. Survey, Bull. 109, pt. 10, p. 153-216. [available online]
Raup, O.B., and Miesch, A.T. (1957) A new method for obtaining significant average directional measurements in cross-stratification studies: Jour. Sed. Petrology, v. 27, p. 313-321.
Reiche, Parry (1938) An analysis of cross-lamination, the Coconino Sandstone: Jour. Geol., v. 46, p. 905-932.
Rubey, W.W., and Bass, N.W. (1925) The geology of Russell County, Kansas: Kansas Geol. Survey Bull. 10, pt. 1, p. 1-86. [available online]
Schoewe, W.H. (1952) Coal resources of the Cretaceous System (Dakota Formation) in central Kansas: Kansas Geol. Survey Bull. 96, pt. 2, p. 69-157. [available online]
Schoewe, W.H., and others (1937) Symposium on the geology, flora, and fauna of "Rock City," a proposed national monument in Ottawa County, Kansas: Kansas Acad. Sci., Trans., v. 40, p. 179-201.
Swineford, Ada (1947) Cemented sandstones of the Dakota and Kiowa formations in Kansas: Kansas Geol. Survey, Bull. 70, pt. 4, p. 53-104. [available online]
Tester, A.C. (1931) The Dakota stage of the type locality: Iowa Geol. Survey, Ann. Rept. (1929) v. 35, p. 195-332.
Thorp, James, and Reed, E.C. (1949) Is there laterite in rocks of the Dakota group?: Science, v. 109, no. 2821, p. 69.
Twenhofel, W.H. (1924) Geology and invertebrate paleontology of the Comanchean and "Dakota" formations of Kansas: Kansas Geol. Survey, Bull. 9, p. 1-135. [available online]
Vector Resultants of Cross-Stratification Dip Bearings in Dakota Sandstone, Ottawa County, Kansas
Kansas Geological Survey, Geology
Placed on web June 7, 2007; originally published in 1959.
Comments to webadmin@kgs.ku.edu
The URL for this page is http://www.kgs.ku.edu/Publications/Bulletins/130_4/index.html