Attributes

Multi-trace Seismic Attributes

• Seismic attributes which are calculated using more than one seismic trace as input
• Provide quantitative information about lateral variations in the seismic data

Seismic Coherence

• Measure of the trace-to-trace similarity of the seismic waveform within a small analysis window
• See Bahorich and Farmer (1995), Marfurt et al. (1998), and Gersztenkorn and Marfurt (1999) for more information about the coherence algorithm.

Dip/Azimuth

• Numerical estimation of the instantaneous dip and azimuth of reflectors within a seismic volume
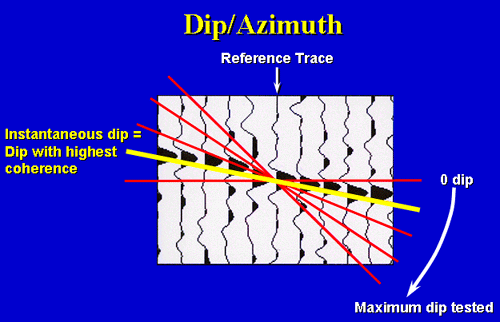
• See Marfurt et al. (1998) for more information about the computation of dip/azimuth.

A schematic 2-D representation of the dip/azimuth calculation. Coherence is calculated for a range of dips (red lines), and in 3-D, azimuths, within a small analysis window around a reference trace. The dip/azimuth combination which has the highest coherence is assigned to be the instantaneous dip/azimuth for the point at the center of the analysis window.

Curvature

Curvature describes how bent a surface is at a particular point and is closely related to the second derivative of the curve defining the surface. The more bent a surface is, the larger its curvature.

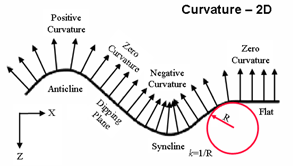
In two dimensions, positive curvature refers to an antiform feature, negative curvature refers to a synform feature, and zero curvature refers to a planar feature.

after Roberts 2001

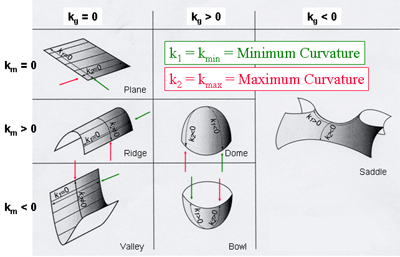
In three dimensions, curvature may be computed at any azimuth about a point, but the most useful curvatures are those defined by planes that are orthogonal to the surface, referred to as normal curvatures. The principal curvatures (maximum curvature and minimum curvature) are one set of normal curvatures.

Maximum Curvature (k_max) - measure of the maximum bending (positive or negative) of the surface at a point

Minimum Curvature (k_min) – curve perpendicular to the maximum curvature

These curvatures can be combined to define other curvature attributes, such as:

Mean Curvature (k_m = (k_max + k_min) / 2) - approximates the average curvature through a point

 

Gaussian Curvature (k_g = k_max*k_min) - indicates whether a surface has been warped or not

 

Curvedness (r = (k²_max + k²_min)^1/2) - measure of intensity of folding

 

Shape Index (s = [(k_max + k _min)/(k_max– k_min)] )– describes local structure as dome, bowl, ridge, plane, etc.

 

after Roberts (2001) and Bergbauer and Pollard (2003)

Other important curvatures include:

 

Most Negative Curvature – most negative value obtained from a search of all possible normal curvatures at a point

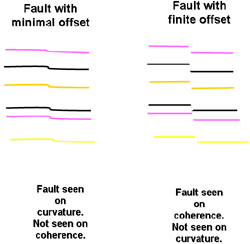
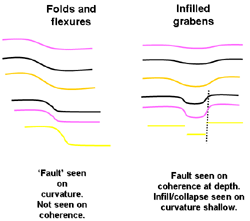
Various curvature attributes have been shown to reveal useful information relating to folds, faults, and lineaments contained within the surface (Roberts, 2001).

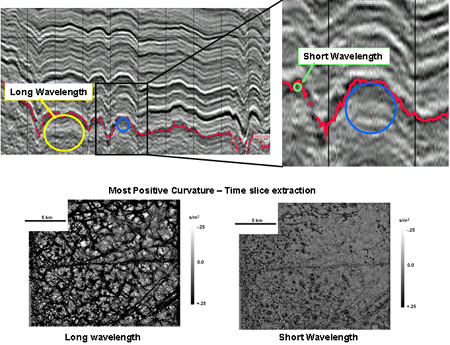
Curvature and rotation are fractal in nature and amenable to multispectral analysis. Long wavelength curvature shows broader features. Short wavelength shows additional detail.

modified from Al-Dossary and Marfut (2005)

Previous workers (e.g., Lisle, 1994; Roberts, 2001) have computed curvature of surfaces interpreted directly from 3-D seismic data whose inline and crossline format create a natural grid. This method suffers from seismic noise and other interpretation problems and treats only a single, well-imaged horizon. Al-Dossary and Marfurt (2005) describe a method of using a moving subvolume to compute curvatures at every point within a 3-D seismic volume. This eliminates interpretation problems and allows us to slice the curvature volumes along time (pseudo depth) or to extract along horizons, thereby allowing us to better image irregular karst features.

Our method